Suppose \( \int \frac{1-7 \cos ^{2} x}{\sin ^{7} x \cos ^{2} x} d x...
Suppose \( \int \frac{1-7 \cos ^{2} x}{\sin ^{7} x \cos ^{2} x} d x=\frac{g(x)}{\sin ^{7} x}+C \), where \( C \) is an arbitrary constant of integration. Then find the value of \( g^{\prime}(0)+g^{\prime \prime}\left(\frac{\pi}{4}\right) \).
📲PW App Link - https://bit.ly/YTAI_PWAP
🌐PW Website - https://www.pw.live
Видео Suppose \( \int \frac{1-7 \cos ^{2} x}{\sin ^{7} x \cos ^{2} x} d x... автора Высшая Математика: Звезды На Небесах Чисел
Видео Suppose \( \int \frac{1-7 \cos ^{2} x}{\sin ^{7} x \cos ^{2} x} d x... автора Высшая Математика: Звезды На Небесах Чисел
Информация
14 января 2025 г. 3:21:59
00:03:54
Похожие видео













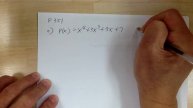
![Баллада о Троице (Garry's Mod) [The Village: Part 3 ] Part #2](http://pic.rutubelist.ru/video/2025-01-14/cc/76/cc7659b6be7ee84484cb21dae165253c.jpg?size=s)